New Scientist is reporting that scientists have created the fastest spinning object ever: a fleck of graphene spun up to an incredible rate of a million rotations per second! Normally, while very cool, that's not the sort of thing I'd write about here. But I had an idle moment, and wondered about what that rotation really meant. I did a little math, and came up with some astonishing numbers.
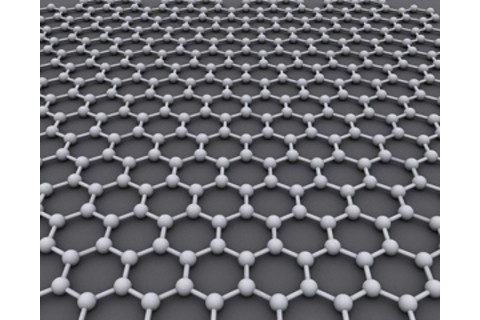
First off, graphene is a flat sheet made up of carbon atoms; each atom connects to the others in a hexagon pattern, and the sheet is only one atom thick! This sheet is incredibly strong, and scientists are excited by it because if it can be produced on a large scale it would have tremendous use. In this case, the scientists created tiny flakes of it only a micron (one-millionth of a meter) across; that's about 1/50th the width of a human hair. They suspended the flakes in a chamber using electric fields, then spun them up using a beam of light. I started picturing what that must be like, these tiny whirling motes of carbon, and realized that the forces on a spinning flake must be huge. And by huge, I mean monstrous. When you spin, you feel a force called the centripetal force. It's what you feel when you're on a merry-go-round, or a car making a turn (it's the same thing as centrifugal force, just seen in a different way). The magnitude of this force, how strong it is, depends on how fast you're moving, and how big a circle you're making. I decided to calculate the size of the force on a flake. As it spins, the corners are making the biggest circles, so they have the highest centripetal force on them. To make things easy, let's assume the distance from the center of the flake to a corner is one micron. We need the velocity of the corner as it spins, which is the distance traveled divided by the time. Well, it makes a complete circle once per one-millionth of a second. And the distance is the circumference of the circle it makes, which is 2 x π x radius. That means: velocity = 2 x π x radius / time = 2 x π x 1 micron / 1 x 10^-6 seconds = 6.3 meters per second That's only about 20 feet/second, or roughly 15 mph. I can bike faster than that. However, remember, we're talking about an object smaller than a human hair! So that's a tremendous speed. The force on it is incredible. Centripetal acceleration* is velocity^2 / radius or (6.3 meters/second)^2 / 1 micron =
4 x 10^7 meter/second^2
If that sounds like a lot, it is: the acceleration of gravity on the Earth's surface is about 10 meter/second^2, so the outwards force you'd feel on that flake would be about four million times the Earth's gravity. Just to give you an idea of how much force that is, I weigh about 170 pounds. If I were under that kind of acceleration, it would feel like hanging from a rope... with a fully loaded oil tanker tied to my feet. Ow. Almost anything on Earth would fly apart at that kind of acceleration, but graphene is just that strong. In fact, the scientists involved said it could withstand even higher speeds! So you can probably get an idea of why this stuff is so cool, and why it might have some interesting applications. I'm thinking roller coasters. You wouldn't even need seat belts to keep people in their seats... but you'd need spatulas to get them off.
^* I switched to acceleration here because it makes the math and the explanation a bit easier. In the end I've been careful about terminology --acceleration and force are related but different -- and I apologize for any confusion.













